• All Notes
• Maths
• Core 2
• Binomial Expansion

Binomial Expansion

• A Bionomial Expansion is a linear polynomial raised to a power, like this (a + b)ⁿ. As n increases, a pattern emerges in the coefficients of each term.

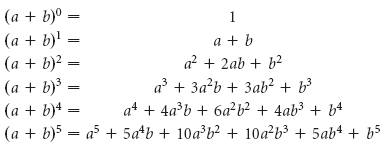
• The coefficients form a pattern called Pascal’s Triangle, where each number is the sum of the two numbers above it.

• For example, (3 + x)³ can be expanded to 1 × 3³ + 3 × 3²x¹ + 3 × 3¹x² + 1 × 3⁰x³ = 27 + 27x + 9x² + x³

• There is another what of calculating the coefficients of each term, using Factorials and the Choose Function. The Choose function is written as ^xC_y, and is calculated by where ‘!’ donates a factorial function (e.g. 5! = 1×2×3×4×5).

• To calculate the coefficient of the zth term of a binomial with exponent n, you would calculate ⁿC_(z-1). For example, the coefficient of the third term in the expansion (a + b)¹¹is ¹¹C₂ = 55 Many calculators have a Choose Function programmed in them. It might look like nCr.